

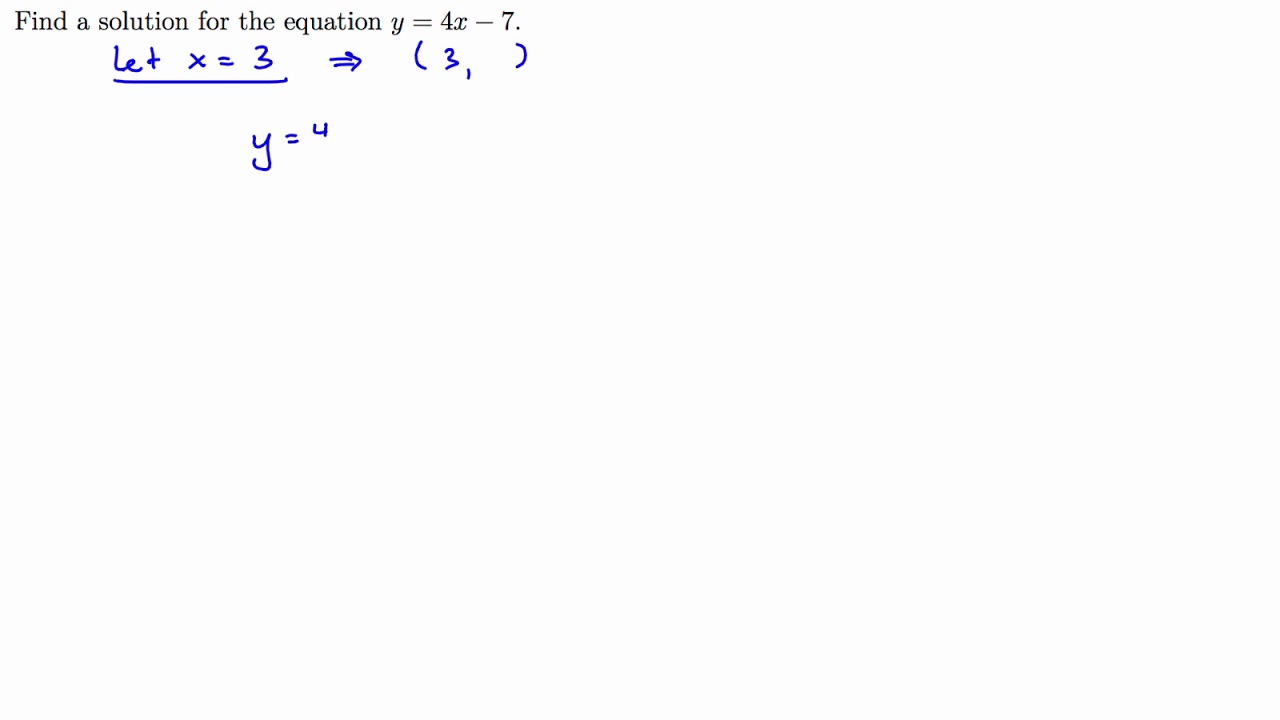
However, there is no single point at which all three planes meet. I set up a matrix and solved and my solution is. Two equations are given and then the solution is shown. In the case below, each plane intersects the other two planes. Im working through an example and my answer is not coming out right. Step 1: First choose two equations and eliminate a variable. The other common example of systems of three variables equations that have no solution is pictured below. Jordi received an inheritance of 12,000 that he divided into three parts and invested in three ways: in a money-market fund paying 3 annual interest in municipal bonds paying 4 annual interest and in mutual funds paying 7 annual interest. A solution to a system of three equations in three variables \left(x,y,z\right),\text Express the solution of a system of dependent equations containing three variables. In order to solve systems of equations in three variables, known as three-by-three systems, the primary goal is to eliminate one variable at a time to achieve back-substitution. Write the equations for a system given a scenario, and solve. Additional Practice Solving a System of Three Equations with Three Variables Step 1 - Solve equation 1 for x: x - y 6 x 6 + y Step 2 - Plug equation 1.Use back substitution to find a solution to a system of three equations.In addition to finding solutions to equations, WolframAlpha also plots the equations and their solutions.
Solving equations yields a solution for the independent variables, either symbolic or numeric. Determine whether an ordered triple is a solution to a system of three equations. Algebraic equations consist of two mathematical quantities, such as polynomials, being equated to each other.So, we add these 2 equations and we get a linear equation with one variable. Step 2: Then substitute the expression for that.

FINDING SOLUTIONS FOR 3 EQUATION SYSTEMS WITH 2 VARIABLES HOW TO
Here is the detailed strategy for how to solve systems of equations with three variables. If we add these 2, we get zero, which means we lose variable y. System of linear equations with three equations in three unknowns can be solved by making two substitutions. 2- Sum both of the equations (forget about the variables for now, work only with their coefficients) 3- After summing both equations and storing the 'newcoefficient' values and assuming you removed x you should have something like: ( (estep1. Notice that we have -3y in the first equation and +3y in the second. 1- Find a number to multiply one of the equations so that you can 'remove' one of the variables. Let’s solve one more system using a different method: So, the solution of this system is (x,y) = (4,1) Now that we found x, we can use it to find y. And there we get a linear equation with one variable x. In the second equation, we write x – 3 instead of y. Let’s the how it works in one simple example:įrom the first equation, we express y using x. Homogeneous Equations 21 Note that the converse of Theorem 1.3.1 is not true: if a homogeneoussystem has nontrivialsolutions, it need not have more variables than equations (the system x1 +x2 0, 2x1 +2x2 0 has nontrivial solutions but m2n. Idea here is to express one variable using the other variable in one equation, and use it in the second equation, where we would get a linear equation with one variable. Here, we will solve systems with 2 variables, given in 2 linear equations. If we have 2 or more linear equations with 2 or more variables, then we have a system of linear equations.


 0 kommentar(er)
0 kommentar(er)
